Stacking Spheres

When you go to a supermarket, you may have seen stacks of fruits, arranged in this pattern. It’s almost like a beehive, in a sixes pattern

Many fragile fruits will bruise up if they are stacked up this - this is why grocers use egg crates for produce like soft apples and pears. 


However, there’s a good reason why grocers stack fruit this way for display.


...and It is not just for the visual appeal...

It's just very self-sturdy.

---

Think of this: imagine a universe made only of perfect, smooth spheres: regular, with all the same size.



Now imagine that each sphere could exert gravity: each sphere was attracted to one another, but were warped in shape due to their attraction to one another. They only have freedom to move around in space.

Unlike the atoms in our universe, there spheres could be attached to any number of spheres that space will allow. These forms will try to take up all available space. They do not overlap each other.

What kind of structure do you think those “spherons” would take – given the choice?

The picture above demonstrates a one-on-top-of-one grid pattern. Each sphere touches up to six neighbors.



The structure results in much of the room not being used. 

It is very difficult to balance two spheres on top of each other if neither of them have surface detail. 

The structure above has room to collapse under its own gravity, should it be disturbed.

My guess is, the spherons would form a one-on-top-of-three nesting pattern.



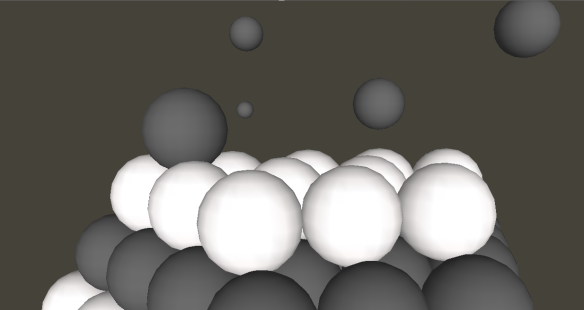
Why? Because In a one-on-top-of-three pattern, the most nested sphere will be in contact with not six, but 12 other spheres. The nested sphere is locked in place by its 12 neighboring spheres. Second-tier neighbors beyond the 12, would be barely peeped at from between the gaps left between the 12 spheres.

In the one-on-top-of-three, the spheres are at rest.

So - that is why grocers stack fruit on top of one another in a one-on-top-of-three position if they can. One, the naturally round shape of many fruits encourages it, and vendors just take advantage of this behavior to save space.

Plus -  it just looks nice. 

---

There is a sequence of numbers called triangular numbers. 

It is a series of numbers that expresses the number of circles it would take to build equilateral triangles layer by layer. 

Equilateral triangles can be built by adding new layers of circles to only one side of the triangle at a time - still allowing every of the dimensions of the shape to grow at the same rate, and thus remain an equilateral.

The exterior shape of perfect spheres allows us to build flat equilateral triangles with them as well.

An equilateral triangle made of spheres is grown by adding a single line of spheres to one side of the triangle - adding one more sphere to each new line of spheres.

Triangular numbers can be used to build regular tetrahedrons.

Tetrahedrons are 3 dimensional shapes that four triangular faces, four corners and six edges.

Like the equilateral triangle, the regular tetrahedron it can be built in only one direction and still keep its regular shape.

Only the tetrahedron requires the addition of equilateral triangles in order to grow.

Stack larger and larger triangles, centered and vertically in one direction, using the one-on-top-of-three pattern for the closest fit, and you should get a regular tetrahedron. 

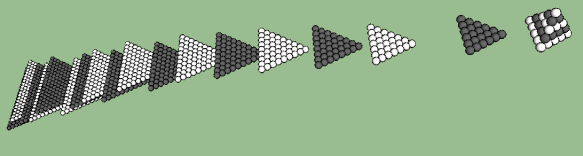
The more layers added to the tetrahedron, the finer the sides and corners of the tetrahedron will be on average.

The 3-dimensional shape with the fewest number of flat sides for any 3-D object. 

"Well, what about spheres?"

Can one really call a sphere a shape with only one side? Several little sides, yes.

In my sight, as long as an object is made up of components - atoms, molecules, magnetic beads, whatever have you - smooth surfaces cannot exist. They can exist as areas, but not objects. 

The only smooth "surface" is a surface with a uniform arrangement of components. But I couldn't say that any physical object can have fewer sides than the components that made it up.

"Then why do you just say you have a tetrahedron? You have made it out of spheres, did you not? You are implying that the spheres are not spheres as well."

Recursive judgement. 

All I can say is this:

Spheres are real. But the laws defining the sphere's dimensions are not based upon the physical attributes of the materials the sphere is made up of.

A sphere is, by definition, the set of points which are all the same distance from a given point in space (Wikipedia).

The problem is, the points are not "real", the way sand grains are real. They are locations in space with no definitions themselves. But physical objects take up volume.

The seen is built from the unseen.

By nature, the concept of the sphere itself is not based on natural laws, but above-natural laws 

The building of the material-based sphere requires above-natural laws. 

If we were to explain a sphere's dimensions based what a physical sphere is made up of, say gravel or marble, doing so would be unexplainable, because the physical components of marble and gravel do not follow the same laws as points - the marble and gravel are not made up of points themselves, but made up of even smaller components. 

But the laws of the points as defined by the sphere are real, strong enough to define what a sphere is for the rest of the universe without wavering from it. If the natural components of a form are unable to form a sphere because its material does not allow a sphere to form, then this is the form's fault and not the sphere's  - for the law that defines the sphere does not change.

And thus are the spiritual laws. 

Why is it that a nihilist can acknowledge the governing laws of physical shapes such as spheres or cubes, which are of such use in this technologically advanced world, (that is, governing laws that are technically non-material based), yet the idea of an non-material God, and any of his governing attributes are "unlikely" - a thought of the imagination? 

If God says that he formed the worlds out of His words, but the "meaningless words and symbols in and of themselves" are used in writing the equations and models that express the unchanging laws of this universe, is this any wonder at all?

Can chaos create science?